Significant Figures (Sig Fig) Calculator


This Significant Figures Calculator calculates the number of significant figures (sig fig), or digits, that a number contains and displays which figures are significant.
Significant figures, or digits, are the values in a number that can be counted on to be accurate. Significant digits in a number are those values which can be known with certainty or a high degree of confidence, while insignificant digits are those which we do not trust as very accurate.
Significant digits are extensively used during measurements. Different measurement tools can record measurements of differing accuracy. Some measurement tools can record much more in detail than other measuring tools. For example, if we have a ruler that only measures centimeters, we can measure to one-hundredth of a meter. If we now change the ruler and get one which now measures millimeters, we can measure to one-thousandth of a meter. Thus, we can have an extra significant digit, because the ruler is more detailed and allows for more accuracy of measurement.
It is important to be honest when making a measurement, so that the resulant value does not appear to be more accurate than the equipment used to make the measurement allows. And how we make the recorded value honest is by controlling the number of digits, or significant figures, used to report the measurement. The recorded value cannot have more significant digits than the measuring tool allows.
So, for example, if we take a measurement and we can state the value is 230 grams. The 2 and 3 are significant digits, while the 0 is not. The 0 is uncertain because we do not trust that it is accurate. Thus, for the number 230, only the 2 and 3 we know with a high degree of accuracy, while the 0 is uncertain and untrusted, so to speak. Thus, the number 230 only has 2 significant digits.
For example, a postage scale measures in grams. It cannot measure a tenth of a gram, a hundredth of a gram, etc, onlyh in grams. It takes a measurement in grams +/- 1 gram. Therefore, it can only measure to the accuracy of 1 significant digit. A two-pan balance, however, can measurement to a one-hundredth of a gram. Therefore, it will have 3 significant digits, one for the ones place, one for the tenth place, and one for the hundredth place. An analytical balance can measure to a thousandth of a gram, so it can have up to 4 significant digits.
However, with all these measurement tools discussed above, the proper significant digits must be adhered to. Imagine if a measurement device can only measure to one-hundredth of a gram such as the two-pan balance and we say that it records a measurement to one-thousandth of a gram. This would make our results very suspicious and it would be inaccurate. If a device can only measure to one-hundredth of a gram, how can you get a value to be as accurate as to one-thousandth of a gram. Therefore, significant figures can be very important in the scientific community. It shows how accurate a number is, based on the number of significant digits present in the number.
Now that you know the importance of significant figures, let's go over the rules for deciding which digits in a number are significant and which are insignificant.
Rules
· All nonzero digits are significant.
Ex. 5.4789- All digits of are nonzero. Therefore, all digits are significant. Thus, it has 5 significant digits.
· Zeros located between nonzero digits are significant.
Ex. 2.00008- All zeroes in this number are significant. Therefore, it has 6 significant digits.
· Zeros at the end of a number are significant if the number contains a decimal.
Ex. 45.00- The zeros in this number are significant. Therefore, it has 4 significant digits.
· Zeros at the end of a number are significant if a decimal point is in place.
Ex. 12000.- The zeros in this number are significant. Therefore, it has 5 significant digits.
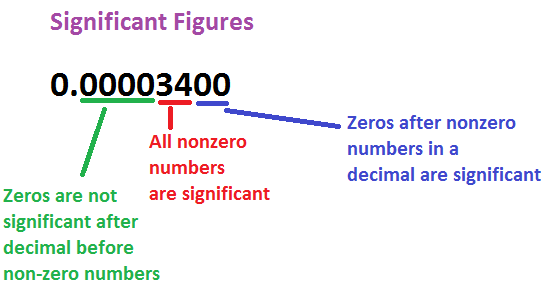
· Zeros at the end of a number are insignificant if if the number does not contain a decimal point.
Ex. 3400- There zeros in this number are not significant. Therefore, it has 2 significant digits.
· Zeros to the left of the first nonzero digit after a decimal point are not sigificant.
Ex. 0.000076- The zeros in this number are not significant. Therefore, it has 2 significant digits.
· The zeros after the first nonzero digit after a decimal point are significant.
Ex. 0.0005600-The first 3 zeros are not significant. The last 2 zeros are. Therefore, this number has 4 significant digits.
These are the general rules for knowing which digits are significant and which aren't.
To use this calculator, a user simply enters in a number for which he wants to find the number of significant figures in the number and which digits of the number are significant. After the number is entered, the user clicks the 'Find Number of Significant Digits' button. The resultant sig fig values will be automatically computed.
Being that electronics, like any other science, deals with measurements, it is important to know how to deal
with significant figures. Depending on the measuring tool in use determines how accurate it can measure. Using the proper number of
significant figures may be extremely important, for the reasons discussed above.
Related Resources
Significant Figures Rounding Calculator
Adding Significant Figures Calculator
Subtracting Significant Figures Calculator
Multiplying Significant Figures Calculator
Dividing Significant Figures Calculator
